tetrahedra torus

Following on from my previous post and prompted by Stan Wagon, I have started to look for other tetrahedral toruses.

It has been mathematically proven that a string of regular tetrahedrons can never make a perfect closed torus* but with a little stretching they cam make a satisfying and useful form.

the following forms were generated by mirror transforming a regular tetrahedron with its four faces coloured yellow, red, green and blue.  the colouring is not arbitrary but a result of each successive transformation.

96 tetrahedral torus with a gap obvious in the upper right corner.

 96 tetrahedral torus with the gap evident.

30 tetrahedral torus, the gap does not look significant but is more clearly seen in the image below.

 30 tetrahedron torus, the gap looks large but this would not need a significant amount of distortion to close.

70 tetrahedron torus

70 tetrahedron torus.  this form does not have a gap but an intersection. the upper centre blue section is intersected by the red section to the right.

80 tetrahedron torus

80 tetrahedron torus illustrating the intersection at the top centre right.

84 tetrahedron torus

84 tetrahedron torus with a gap in the chain at the back left.  the gap is less than one tetrahedron.

 *Stanislaw Swierczkowski 1959, On chains of regular tetrahedra. reference courtesy of Stan Wagon. 

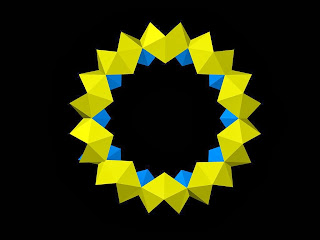
96 tetrahelix torus

A paper model of a torus made from 96 tetrahedrons.
There is a gap equivalent to less than one tetrahedron which can be bridged with some minor deformation.
Thanks to Stan Wagon for bringing this problem to my attention.
This was made as a model for a tensegrity structure which is on my long term to do list and was not intended to be posted just yet.

hexagonal tensegrity grid structure

this hexagonal grid structure can be viewed as a lattice of isolated hexagons which fold over and under each other without touching.

when viewed as a tensegrity it is a very simple structure made up of left and right handed 3 strut prisms.

as the hexagonal cells do not touch each other, they are 'islands of compression in a sea of tension' to quote Buckminster Fuller.

this grid is sitting on a flat surface although it looks curved.

form this angle it is easier to see that the hexagonal cells are separate.

this structure may be useful as a model for certain types cellular biology or crystal structures.

the hexagonal cells are made from compression rods but they are not rigid forms, each joint connection forming the hexagon is a pin joint which is free to swivel on all axis.

It is the connecting tensile strings which hold the hexagonal cell shapes together but also keeps each cell apart.

the grid can easily be deformed into a dome shape.  due to the nature of tensegrity, the tensile forces in the assembly are contained and neutralised at the cellular level unlike traditional structures where the tensile forces are aggregated and transferred in total to the perimeter (or ground) to maintain rigidity.

This obviates the need for a continuous perimeter compression ring which is an essential part of current cable dome structures.

www tower

the www tower is a tensegrity structure made from three continuous over lapping 'www' shaped beams which are separated but held together by tension cables.

the structure is built from rigid compression members held together by pin joints and isolated by tension cables.  the greater the tension in the cables, the more rigid the overall structure becomes with an increased capacity to carry load.

the tower structure is similar to the lattice slab structures developed by Reno Motro.

the structure can be easily assembled or collapsed as required and can be utilised for portable or deployable structures.  the structure also has the potential to be articulated like a prehensile tail by using actuators to vary the tensioning in each line.

this type of structure can be viewed at both the micro and macro scale.  it could be used for large scale building structures or at the micro level, compressive members in a tensegrity structure - of this or any other configuration - could be decomposed into an assembly of smaller and smaller compressive members.

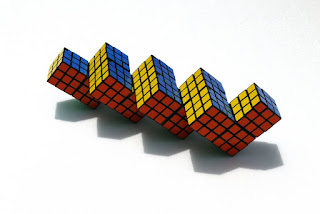
Cubic Helix

this is a cubic helix
as I couldn't find any images of a cubic helix on line I thought I'd post some.

the helix is made from 12 mini Rubik's cubes stuck together with double sided tape.

the cubic helix maybe a very simple form but for some reason it is still quite difficult to comprehend and it has an illusionary quality to it, almost like some of Escher's stair drawings.

tensegrity tetrahelix

Here are some images of a tetrahelix made with a tensegrity structure.  The basic structure is what is often referred to as v-expander modules (not sure of their origin..).

The tetrahedron modules were assembled by a mirror and rotation transformation, in an attempt to make the structure more random (but it doesn't appear to have worked.  the next model will be made up of regular 'boat' modules (blocks of 3 tetrahedrons).

suspended over the pool at our house.

looking down roughly on axis

a close up of the tendon used to stress the structure.  the orange tendon holds the form together, cut the tendon and the form collapses, analogous with deflating a tyre.  as a note tensegrity and pneumatics are conceptually very similar.

a basic v-expander module, rational and very beautiful - to my eyes anyway.