tetrahedra torus

Following on from my previous post and prompted by Stan Wagon, I have started to look for other tetrahedral toruses.

It has been mathematically proven that a string of regular tetrahedrons can never make a perfect closed torus* but with a little stretching they cam make a satisfying and useful form.

the following forms were generated by mirror transforming a regular tetrahedron with its four faces coloured yellow, red, green and blue.  the colouring is not arbitrary but a result of each successive transformation.

96 tetrahedral torus with a gap obvious in the upper right corner.

 96 tetrahedral torus with the gap evident.

30 tetrahedral torus, the gap does not look significant but is more clearly seen in the image below.

 30 tetrahedron torus, the gap looks large but this would not need a significant amount of distortion to close.

70 tetrahedron torus

70 tetrahedron torus.  this form does not have a gap but an intersection. the upper centre blue section is intersected by the red section to the right.

80 tetrahedron torus

80 tetrahedron torus illustrating the intersection at the top centre right.

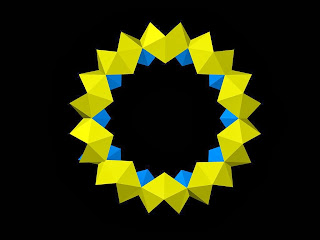
84 tetrahedron torus

84 tetrahedron torus with a gap in the chain at the back left.  the gap is less than one tetrahedron.

 *Stanislaw Swierczkowski 1959, On chains of regular tetrahedra. reference courtesy of Stan Wagon.